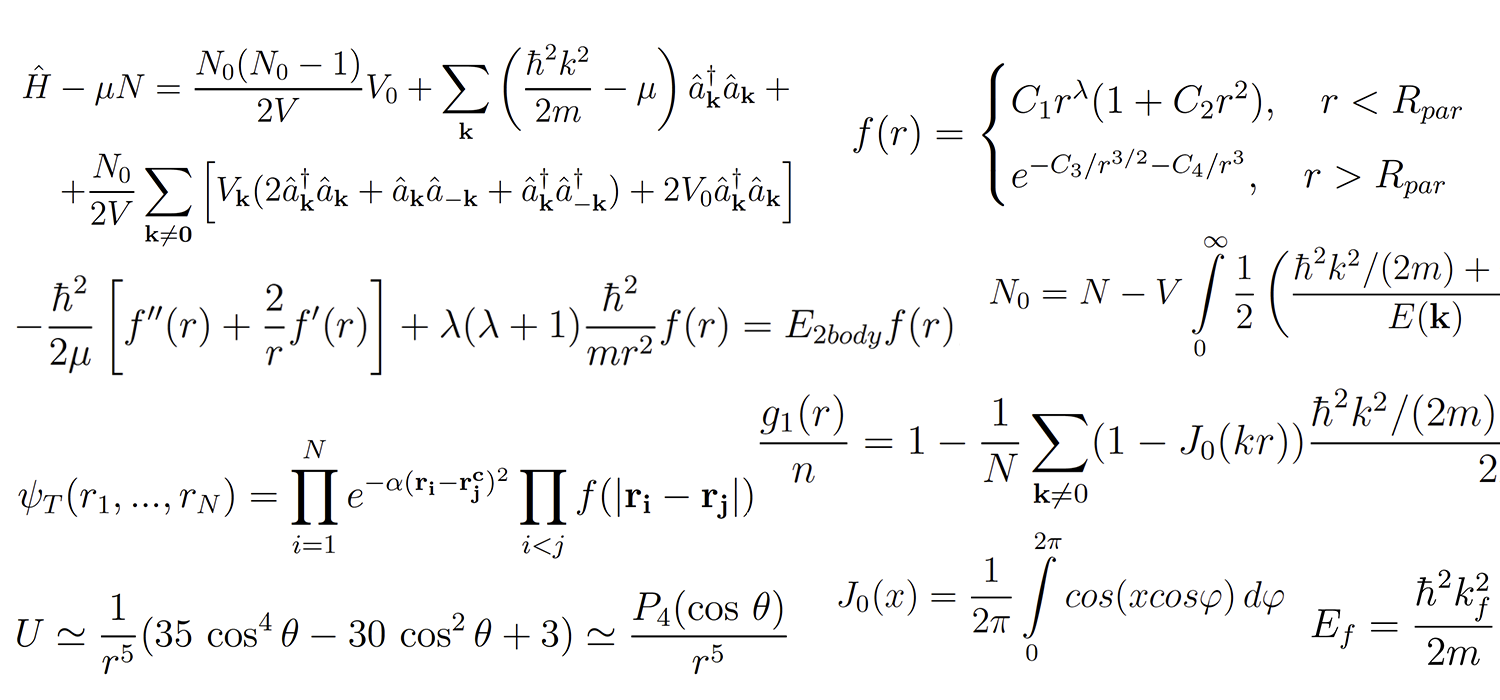
Meine Spezialität ist die Physik der kondensierten Materie. Natürlich ist es beim Eintauchen erforderlich, viele wissenschaftliche Artikel zu studieren, aber es kann viel Zeit in Anspruch nehmen, mindestens einen zu analysieren. Pro Monat werden mehr als tausend Artikel auf
arxiv im Bereich
cond-mat veröffentlicht . Es gibt eine Situation, in der viele Forscher, insbesondere Anfänger, keine ganzheitliche Sicht auf ihr Wissenschaftsgebiet haben. Das in diesem Artikel beschriebene Tool fasst den Inhalt der Datenbank wissenschaftlicher Artikel zusammen und soll die Arbeit mit Literatur beschleunigen.
Es ist erwähnenswert, dass wir ein Fahrrad erfinden, nur unser Fahrrad wird kostenlos fahren (die Preise für
bezahlte Analoga sind so unanständig, dass sie nicht als Hinweis akzeptiert werden).
Das Fahrrad wird in Python zusammengebaut,
Gensim wird für die thematische Modellierung verwendet und
Plot.ly wird für die Visualisierung verwendet. Am Ende des Artikels befinden sich Links zu Jupyter-Laptops und GitHub.
Das Ausgangsmaterial für die Arbeit sind Anmerkungen und Texte wissenschaftlicher Artikel. Wenn erstere uns in einer „vorgefertigten“ XML-Form zur Verfügung stehen, müssen die PDF-Dateien der Artikel in txt konvertiert werden. Aufgrund dieses Prozesses verbleibt viel „Müll“ in den Texten, sodass sie ernsthaft gereinigt werden müssen.
Beispielanmerkung<?xml version="1.0" encoding="UTF-8"?> <feed xmlns="http://www.w3.org/2005/Atom"> <link href="http://arxiv.org/api/query?search_query%3D%26id_list%3D1706.09062%26start%3D0%26max_results%3D10" rel="self" type="application/atom+xml"/> <title type="html">ArXiv Query: search_query=&id_list=1706.09062&start=0&max_results=10</title> <id>http://arxiv.org/api/ECEwpFhuO79sa+LzMzx6/iStFak</id> <updated>2018-05-01T00:00:00-04:00</updated> <opensearch:totalResults xmlns:opensearch="http://a9.com/-/spec/opensearch/1.1/">1</opensearch:totalResults> <opensearch:startIndex xmlns:opensearch="http://a9.com/-/spec/opensearch/1.1/">0</opensearch:startIndex> <opensearch:itemsPerPage xmlns:opensearch="http://a9.com/-/spec/opensearch/1.1/">10</opensearch:itemsPerPage> <entry> <id>http://arxiv.org/abs/1706.09062v1</id> <updated>2017-06-27T22:03:24Z</updated> <published>2017-06-27T22:03:24Z</published> <title>On Bose-Einstein condensation and superfluidity of trapped photons with coordinate-dependent mass and interactions</title> <summary> The condensate density profile of trapped two-dimensional gas of photons in an optical microcavity, filled by a dye solution, is analyzed taking into account a coordinate-dependent effective mass of cavity photons and photon-photon coupling parameter. The profiles for the densities of the superfluid and normal phases of trapped photons in the different regions of the system at the fixed temperature are analyzed. The radial dependencies of local mean-field phase transition temperature $T_c^0 (r)$ and local Kosterlitz-Thouless transition temperature $T_c (r)$ for trapped microcavity photons are obtained. The coordinate dependence of cavity photon effective mass and photon-photon coupling parameter is important for the mirrors of smaller radius with the high trapping frequency, which provides BEC and superfluidity for smaller critical number of photons at the same temperature. We discuss a possibility of an experimental study of the density profiles for the normal and superfluid components in the system under consideration. </summary> <author> <name>Oleg L. Berman</name> </author> <author> <name>Roman Ya. Kezerashvili</name> </author> <author> <name>Yurii E. Lozovik</name> </author> <arxiv:doi xmlns:arxiv="http://arxiv.org/schemas/atom">10.1364/JOSAB.34.001649</arxiv:doi> <link title="doi" href="http://dx.doi.org/10.1364/JOSAB.34.001649" rel="related"/> <arxiv:comment xmlns:arxiv="http://arxiv.org/schemas/atom">14 page 5, figures</arxiv:comment> <link href="http://arxiv.org/abs/1706.09062v1" rel="alternate" type="text/html"/> <link title="pdf" href="http://arxiv.org/pdf/1706.09062v1" rel="related" type="application/pdf"/> <arxiv:primary_category xmlns:arxiv="http://arxiv.org/schemas/atom" term="cond-mat.mes-hall" scheme="http://arxiv.org/schemas/atom"/> <category term="cond-mat.mes-hall" scheme="http://arxiv.org/schemas/atom"/> </entry> </feed>
BeispieltextOn Bose-Einstein condensation and super¬‚uidity of trapped photons with
coordinate-dependent mass and interactions
Oleg L. Berman1,2, Roman Ya. Kezerashvili1,2, and Yurii E. Lozovik3,4
1Physics Department, New York City College of Technology, The City University of New York,
2The Graduate School and University Center, The City University of New York,
Brooklyn, NY 11201, USA
3Institute of Spectroscopy, Russian Academy of Sciences, 142190 Troitsk, Moscow, Russia
4National Research University Higher School of Economics, Moscow, Russia
New York, NY 10016, USA
(Dated: June 29, 2017)
The condensate density pro¬Ѓle of trapped two-dimensional gas of photons in an optical micro-
cavity, ¬Ѓlled by a dye solution, is analyzed taking into account a coordinate-dependent e¬Ђective
mass of cavity photons and photon-photon coupling parameter. The pro¬Ѓles for the densities of the
super¬‚uid and normal phases of trapped photons in the di¬Ђerent regions of the system at the ¬Ѓxed
temperature are analyzed. The radial dependencies of local mean-¬Ѓeld phase transition temperature
T 0
c (r) and local Kosterlitz-Thouless transition temperature Tc(r) for trapped microcavity photons
are obtained. The coordinate dependence of cavity photon e¬Ђective mass and photon-photon cou-
pling parameter is important for the mirrors of smaller radius with the high trapping frequency,
which provides BEC and super¬‚uidity for smaller critical number of photons at the same temper-
ature. We discuss a possibility of an experimental study of the density pro¬Ѓles for the normal and
super¬‚uid components in the system under consideration.
Key words: Photons in a microcavity; Bose-Einstein condensation of photons; super¬‚uidity of
photons.
PACS numbers: 03.75.Hh, 42.55.Mv, 67.85.Bc, 67.85.Hj
I.
INTRODUCTION
When a system of bosons is cooled to low temperatures, a substantial fraction of the particles spontaneously occupy
the single lowest energy quantum state. This phenomenon is known as Bose-Einstein condensation (BEC) and its
occurs in many-particle systems of bosons with masses m and temperature T when the de Broglie wavelength of the
Bose particle exceeds the mean interparticle distance [1]. The most remarkable consequence of BEC is that there
should be a temperature below which a ¬Ѓnite fraction of all the bosons ЂњcondenseЂќ into the same one-particle state
with macroscopic properties described by a single condensate wavefunction, promoting quantum physics to classical
time- and length scales.
Most recently, the observations at room temperature of the BEC of two-dimensional photon gas con¬Ѓned in an optical
microcavity, formed by spherical mirrors and ¬Ѓlled by a dye solution, were reported [2Ђ“5]. The interaction between
microcavity photons is achieved through the interaction of the photons with the non-linear media of a microcavity,
¬Ѓlled by a dye solution. While the main contribution to the interaction in the experiment, reported in Ref. 2, is
thermooptic, it is not a contact interaction.
It is known that BEC for bosons can exist without particle-particle
interactions [6] (see Ref. 1 for the details), but at least the interactions with the surrounding media are necessary to
achieve thermodynamical equilibrium. For photon BEC it can be achieved by interaction with incoherent phonons [7].
The in¬‚uence of interactions on condensate-number ¬‚uctuations in a BEC of microcavity photons was studied in Ref. 8.
The kinetics of photon thermalization and condensation was analyzed in Refs. 9Ђ“11. The kinetics of trapped photon
gas in a microcavity, ¬Ѓlled by a dye solution, was studied, and, a crossover between driven-dissipative system laser
dynamics and a thermalized Bose-Einstein condensation of photons was observed [12].
In previous theoretical studies the equation of motion for a BEC of photons con¬Ѓned by the axially symmetrical
trap in a microcavity was obtained.
It was assumed that the changes of the cavity width are much smaller than
the width of the trap [13]. This assumption results in the coordinate-independent e¬Ђective photon mass mph and
photon-photon coupling parameter g. In this Paper, we study the local super¬‚uid and normal density pro¬Ѓles for
trapped two-dimensional gas of photons with the coordinate-dependent e¬Ђective mass and photon-photon coupling
parameter in a an optical microcavity, ¬Ѓlled by a dye solution. We propose the approach to study the local BEC
and local super¬‚uidity of cavity photon gas in the framework of local density approximation (LDA) in the traps of
larger size without the assumption, that total changes of the cavity width are much smaller than the size of the trap.
In this case, we study the e¬Ђects of coordinate-dependent e¬Ђective mass and photon-photon coupling parameter on
the super¬‚uid and normal density pro¬Ѓles as well as the pro¬Ѓles of the local temperature of the phase transition for
trapped cavity photons. Such approach is useful for the mirrors of smaller radius with the high trapping frequency,
2
which provide BEC and super¬‚uidity for smaller critical number of photons at the same temperature.
The paper is organized in the following way.
In Sec. II, we obtain the condensate density pro¬Ѓle for trapped
microcavity photon BEC with locally variable mass and interactions. The expression for the number of particles in a
condensate is analyzed in Sec. III. In Sec. IV, the dependence of the condensate parameters on the geometry of the
trap is discussed. In Sec. V, we study the collective excitation spectrum and super¬‚uidity of 2D weakly-interacting
Bose gas of cavity photons. The results of our calculations are discussed in Sec. VI. The proposed experiment for
measuring the distribution of the local density of a photon BEC is described in Sec. VII. The conclusions follow in
Sec. VIII.
II. THE CONDENSATE DENSITY PROFILE
While at ¬Ѓnite temperatures there is no true BEC in any in¬Ѓnite untrapped two-dimensional (2D) system, a true
2D BEC quantum phase transition can be obtained in the presence of a con¬Ѓning potential [14, 15]. In an in¬Ѓnite
translationally invariant two-dimensional system, without a trap, super¬‚uidity occurs via a Kosterlitz€'Thouless
super¬‚uid (KTS) phase transition [16]. While KTS phase transition occurs in systems, characterized by thermal
equilibrium, it survives in a dissipative highly nonequilibrium system driven into a steady state [17].
The trap for the cavity photons can be formed by the concave spherical mirrors of the microcavity, that provide
the axial symmetry for a trapped gas of photons. Thus the transverse (along xy plane of the cavity) con¬Ѓnement
of photons can be achieved by using an optical microcavity with a variable width. Let us introduce the frame of
reference, where z€'axis is directed along the axis of cavity mirrors, and (x, y) plane is perpendicular to this axis. The
energy spectrum E(k) for small wave vectors k of photons, con¬Ѓned in z direction in an ideal microcavity with the
coordinate-dependent width L(r), is given by [2]
E(k) =
ЇhЂcњn
...
[23] L. Onsager, ЂњStatistical Hydrodynamics,Ђќ Nuovo Cimento Suppl. 6, 279 (1949).
[24] RP Feynman, ЂњApplication of Quantum Mechanics to Liquid Helium,Ђќ Prog. Low Temp. Phys. 1, 17 (1955).
[25] PC Hohenberg and PC Martin, ЂњMicroscopic Theory of Super¬‚uid Helium,Ђќ Ann. Phys. 34, 291 (1965).
[26] G. Blatter, MY FeigelЂman, YB Geshkenbein, AI Larkin, and VM Vinokur, ЂњVortices in high-temperature super-
conductors,Ђќ Rev. Mod. Phys. 66, 1125 (1994).
[27] NS Voronova and Yu. E. Lozovik, ЂњExcitons in cores of exciton-polariton vortices,Ђќ Phys. Rev. B 86, 195305 (2012);
NS Voronova, AA Elistratov, and Yu. E. Lozovik, ЂњDetuning-Controlled Internal Oscillations in an Exciton-Polariton
Condensate,Ђќ Phys. Rev. Lett. 115, 186402 (2015) .
[28] A. Gri¬ѓn, ЂњConserving and gapless approximations for an inhomogeneous Bose gas at ¬Ѓnite temperatures,Ђќ Phys. Rev. B
53, 9341 (1996).
[29] AA Abrikosov, LP Gorkov and IE Dzyaloshinski, Methods of Quantum Field Theory in Statistical Physics (Prentice-
Hall, Englewood Cli¬Ђs. NJ, 1963).
[30] OL Berman, Yu. E. Lozovik, and DW Snoke, ЂњTheory of Bose-Einstein condensation and super¬‚uidity of two-
dimensional polaritons in an in-plane harmonic potential,Ђќ Phys. Rev. B 77, 155317 (2008).
[31] OL Berman, R. Ya. Kezerashvili, and K. Ziegler, ЂњSuper¬‚uidity and collective properties of excitonic polaritons in gapped
graphene in a microcavityЂќ, Phys. Rev. B 86, 235404 (2012).
[32] A. Amo, J. Lefr`ere, S. Pigeon, C. Adrados, C. Ciuti, I. Carusotto, R. Houdrґe, E. Giacobino, and A. Bramati, ЂњSuper¬‚uidity
of polaritons in semiconductor microcavities,Ђќ Nature Physics 5, 805 (2009).
[33] JP Fernґandez and WJ Mullin, ЂњThe Two-Dimensional Bose€'Einstein Condensate,Ђќ J. Low. Temp. Phys. 128, 233
Von Anmerkungen benötigen wir
nur Informationen über Autoren und Unterabschnitte von Artikeln.
Im Folgenden betrachten wir als Beispiel eine Reihe von Artikeln aus dem Bereich Cond-Mat für das 2017. Jahr. Alles, was beschrieben wird, ist für jeden anderen Abschnitt leicht zu reproduzieren.
Der einfachste Weg, um mit dem Studium von Texten zu beginnen, besteht darin, eine Liste der für uns interessanten Schlüsselwörter zu erstellen und den Anteil der Artikel zu berechnen, in denen sie erscheinen.
Lassen Sie uns zum Beispiel die Veränderung der Aktie im Vergleich zu 2010 bewerten.
Die Differenz der Aktien für das 2017. und 2010. Jahr. (Anmerkung: Der Nobelpreis für Physik 2016 wurde für die Untersuchung der topologischen Phasen der Materie verliehen.)Basierend auf dem Inhalt der Texte erstellen wir außerdem das
word2vec- Modell (zur Visualisierung ist es besser, ein breiteres Fenster mit 20 Wörtern zu verwenden). Für jeden Schlüssel nehmen wir N nächste Nachbarn und berechnen mit Hilfe von t-SNE deren 2D-Koordinaten. Wir untersuchen, wie Schlüsselwörter miteinander in Beziehung stehen:
Wolke von Schlüsselwörtern und ihren Satelliten, N = 100. Je weiter der N-te Nachbar entfernt ist, desto stärker wird das Wort hervorgehoben. Relevante Paare: Graphen + Halbleiter, Qubit + Punkt, Topologisch + Hall, Bose + KondensationAuf arxiv gibt es Unterabschnitte für jeden Abschnitt. Wir finden heraus, in welchen Unterabschnitten die Schlüsselwörter gefunden werden:
Dekodierung der Namen von Unterteilungen von cond-matIm obigen Abschnitt wird die Arbeit mit einer Reihe von Schlüsselwörtern beschrieben, die Sie manuell erstellen möchten. Einige Themen wurden jedoch möglicherweise übersprungen. Lassen Sie uns ein
LDA- Modell erstellen und die Themen betrachten:
Für jedes Thema erhalten wir eine Liste der entsprechenden Artikel:
Wie Sie wissen, ist es beim Lesen von Artikeln immer nützlich, die Links zu studieren. Können wir einige Informationen über sie sammeln? Wir können! Schauen wir uns den Ende des Textes an.
Schwanz[23] L. Onsager, ЂњStatistical Hydrodynamics,Ђќ Nuovo Cimento Suppl. 6, 279 (1949).
[24] RP Feynman, ЂњApplication of Quantum Mechanics to Liquid Helium,Ђќ Prog. Low Temp. Phys. 1, 17 (1955).
[25] PC Hohenberg and PC Martin, ЂњMicroscopic Theory of Super¬‚uid Helium,Ђќ Ann. Phys. 34, 291 (1965).
[26] G. Blatter, MY FeigelЂman, YB Geshkenbein, AI Larkin, and VM Vinokur, ЂњVortices in high-temperature super-
conductors,Ђќ Rev. Mod. Phys. 66, 1125 (1994).
[27] NS Voronova and Yu. E. Lozovik, ЂњExcitons in cores of exciton-polariton vortices,Ђќ Phys. Rev. B 86, 195305 (2012);
NS Voronova, AA Elistratov, and Yu. E. Lozovik, ЂњDetuning-Controlled Internal Oscillations in an Exciton-Polariton
Condensate,Ђќ Phys. Rev. Lett. 115, 186402 (2015) .
[28] A. Gri¬ѓn, ЂњConserving and gapless approximations for an inhomogeneous Bose gas at ¬Ѓnite temperatures,Ђќ Phys. Rev. B
53, 9341 (1996).
[29] AA Abrikosov, LP Gorkov and IE Dzyaloshinski, Methods of Quantum Field Theory in Statistical Physics (Prentice-
Hall, Englewood Cli¬Ђs. NJ, 1963).
[30] OL Berman, Yu. E. Lozovik, and DW Snoke, ЂњTheory of Bose-Einstein condensation and super¬‚uidity of two-
dimensional polaritons in an in-plane harmonic potential,Ђќ Phys. Rev. B 77, 155317 (2008).
[31] OL Berman, R. Ya. Kezerashvili, and K. Ziegler, ЂњSuper¬‚uidity and collective properties of excitonic polaritons in gapped
graphene in a microcavityЂќ, Phys. Rev. B 86, 235404 (2012).
[32] A. Amo, J. Lefr`ere, S. Pigeon, C. Adrados, C. Ciuti, I. Carusotto, R. Houdrґe, E. Giacobino, and A. Bramati, ЂњSuper¬‚uidity
of polaritons in semiconductor microcavities,Ђќ Nature Physics 5, 805 (2009).
[33] JP Fernґandez and WJ Mullin, ЂњThe Two-Dimensional Bose€'Einstein Condensate,Ђќ J. Low. Temp. Phys. 128, 233
PDF Converter verarbeitet einen Abschnitt mit einer Bibliographie gut. Dies bedeutet, dass Links mit wenigen regulären Ausdrücken abgerufen werden können. Als Ergebnis erhalten wir eine Liste häufig zitierter, unbedingt zu lesender Artikel.
Schauen Sie sich diese Links in
Google Scholar an :
Erstellen Sie eine Liste der aktivsten Autoren für jeden Unterabschnitt - wir extrahieren und berechnen den Inhalt des Autoren-Tags aus Anmerkungen. Die Anzahl der von einem bestimmten Autor veröffentlichten Artikel ist ein verständliches, aber unzuverlässiges Merkmal. Sie kann durch den Median der Anzahl der Mitautoren ergänzt werden (siehe Notizbücher).
Autoren aus mes-hall sind konkurrenzlos: Ihr durchschnittliches Arbeitstempo beträgt mehr als einen Artikel pro Woche ...Schließlich schätzen wir den Anteil der Unterabschnitte:
Demo-Laptops:
cond-mat.17 ,
astro-ph.17 ,
cs.17 ,
math.17Github :
ilovescience