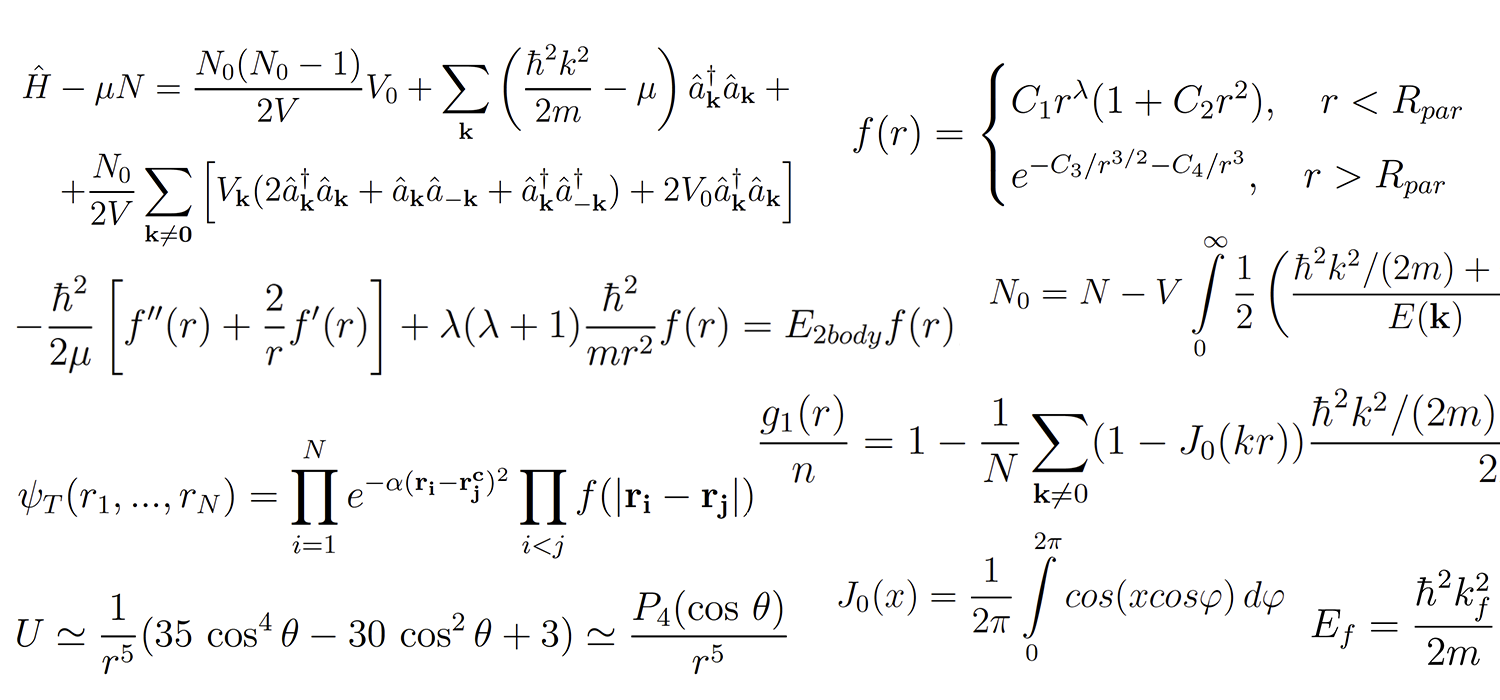
Mi especialidad es la física de la materia condensada. Por supuesto, en el proceso de inmersión en él, se requiere estudiar muchos artículos científicos, pero puede tomar mucho tiempo analizar al menos uno. Se publican más de mil artículos por mes en
arxiv en la sección
cond . Existe una situación en la que muchos investigadores, especialmente los principiantes, no tienen una visión holística de su campo de la ciencia. La herramienta descrita en este artículo resume los contenidos de la base de datos de artículos científicos y está diseñada para acelerar el trabajo con la literatura.
Vale la pena mencionar que estamos inventando una bicicleta, solo la nuestra irá gratis (los precios de
los análogos pagados son tan indecentes que no se aceptan para indicar).
La bicicleta se ensamblará en Python,
Gensim se usa para modelado temático y
Plot.ly se usa para visualización. Al final del artículo hay enlaces a las computadoras portátiles Jupyter y GitHub.
El material fuente para el trabajo son anotaciones y textos de artículos científicos. Si los primeros están disponibles para nosotros en un formato XML "listo para usar", entonces los archivos PDF de los artículos deben convertirse a txt, como resultado de este proceso queda mucha "basura" en los textos, por lo que deben limpiarse seriamente.
Anotación de muestra<?xml version="1.0" encoding="UTF-8"?> <feed xmlns="http://www.w3.org/2005/Atom"> <link href="http://arxiv.org/api/query?search_query%3D%26id_list%3D1706.09062%26start%3D0%26max_results%3D10" rel="self" type="application/atom+xml"/> <title type="html">ArXiv Query: search_query=&id_list=1706.09062&start=0&max_results=10</title> <id>http://arxiv.org/api/ECEwpFhuO79sa+LzMzx6/iStFak</id> <updated>2018-05-01T00:00:00-04:00</updated> <opensearch:totalResults xmlns:opensearch="http://a9.com/-/spec/opensearch/1.1/">1</opensearch:totalResults> <opensearch:startIndex xmlns:opensearch="http://a9.com/-/spec/opensearch/1.1/">0</opensearch:startIndex> <opensearch:itemsPerPage xmlns:opensearch="http://a9.com/-/spec/opensearch/1.1/">10</opensearch:itemsPerPage> <entry> <id>http://arxiv.org/abs/1706.09062v1</id> <updated>2017-06-27T22:03:24Z</updated> <published>2017-06-27T22:03:24Z</published> <title>On Bose-Einstein condensation and superfluidity of trapped photons with coordinate-dependent mass and interactions</title> <summary> The condensate density profile of trapped two-dimensional gas of photons in an optical microcavity, filled by a dye solution, is analyzed taking into account a coordinate-dependent effective mass of cavity photons and photon-photon coupling parameter. The profiles for the densities of the superfluid and normal phases of trapped photons in the different regions of the system at the fixed temperature are analyzed. The radial dependencies of local mean-field phase transition temperature $T_c^0 (r)$ and local Kosterlitz-Thouless transition temperature $T_c (r)$ for trapped microcavity photons are obtained. The coordinate dependence of cavity photon effective mass and photon-photon coupling parameter is important for the mirrors of smaller radius with the high trapping frequency, which provides BEC and superfluidity for smaller critical number of photons at the same temperature. We discuss a possibility of an experimental study of the density profiles for the normal and superfluid components in the system under consideration. </summary> <author> <name>Oleg L. Berman</name> </author> <author> <name>Roman Ya. Kezerashvili</name> </author> <author> <name>Yurii E. Lozovik</name> </author> <arxiv:doi xmlns:arxiv="http://arxiv.org/schemas/atom">10.1364/JOSAB.34.001649</arxiv:doi> <link title="doi" href="http://dx.doi.org/10.1364/JOSAB.34.001649" rel="related"/> <arxiv:comment xmlns:arxiv="http://arxiv.org/schemas/atom">14 page 5, figures</arxiv:comment> <link href="http://arxiv.org/abs/1706.09062v1" rel="alternate" type="text/html"/> <link title="pdf" href="http://arxiv.org/pdf/1706.09062v1" rel="related" type="application/pdf"/> <arxiv:primary_category xmlns:arxiv="http://arxiv.org/schemas/atom" term="cond-mat.mes-hall" scheme="http://arxiv.org/schemas/atom"/> <category term="cond-mat.mes-hall" scheme="http://arxiv.org/schemas/atom"/> </entry> </feed>
Texto de muestraOn Bose-Einstein condensation and super¬‚uidity of trapped photons with
coordinate-dependent mass and interactions
Oleg L. Berman1,2, Roman Ya. Kezerashvili1,2, and Yurii E. Lozovik3,4
1Physics Department, New York City College of Technology, The City University of New York,
2The Graduate School and University Center, The City University of New York,
Brooklyn, NY 11201, USA
3Institute of Spectroscopy, Russian Academy of Sciences, 142190 Troitsk, Moscow, Russia
4National Research University Higher School of Economics, Moscow, Russia
New York, NY 10016, USA
(Dated: June 29, 2017)
The condensate density pro¬Ѓle of trapped two-dimensional gas of photons in an optical micro-
cavity, ¬Ѓlled by a dye solution, is analyzed taking into account a coordinate-dependent e¬Ђective
mass of cavity photons and photon-photon coupling parameter. The pro¬Ѓles for the densities of the
super¬‚uid and normal phases of trapped photons in the di¬Ђerent regions of the system at the ¬Ѓxed
temperature are analyzed. The radial dependencies of local mean-¬Ѓeld phase transition temperature
T 0
c (r) and local Kosterlitz-Thouless transition temperature Tc(r) for trapped microcavity photons
are obtained. The coordinate dependence of cavity photon e¬Ђective mass and photon-photon cou-
pling parameter is important for the mirrors of smaller radius with the high trapping frequency,
which provides BEC and super¬‚uidity for smaller critical number of photons at the same temper-
ature. We discuss a possibility of an experimental study of the density pro¬Ѓles for the normal and
super¬‚uid components in the system under consideration.
Key words: Photons in a microcavity; Bose-Einstein condensation of photons; super¬‚uidity of
photons.
PACS numbers: 03.75.Hh, 42.55.Mv, 67.85.Bc, 67.85.Hj
I.
INTRODUCTION
When a system of bosons is cooled to low temperatures, a substantial fraction of the particles spontaneously occupy
the single lowest energy quantum state. This phenomenon is known as Bose-Einstein condensation (BEC) and its
occurs in many-particle systems of bosons with masses m and temperature T when the de Broglie wavelength of the
Bose particle exceeds the mean interparticle distance [1]. The most remarkable consequence of BEC is that there
should be a temperature below which a ¬Ѓnite fraction of all the bosons ЂњcondenseЂќ into the same one-particle state
with macroscopic properties described by a single condensate wavefunction, promoting quantum physics to classical
time- and length scales.
Most recently, the observations at room temperature of the BEC of two-dimensional photon gas con¬Ѓned in an optical
microcavity, formed by spherical mirrors and ¬Ѓlled by a dye solution, were reported [2Ђ“5]. The interaction between
microcavity photons is achieved through the interaction of the photons with the non-linear media of a microcavity,
¬Ѓlled by a dye solution. While the main contribution to the interaction in the experiment, reported in Ref. 2, is
thermooptic, it is not a contact interaction.
It is known that BEC for bosons can exist without particle-particle
interactions [6] (see Ref. 1 for the details), but at least the interactions with the surrounding media are necessary to
achieve thermodynamical equilibrium. For photon BEC it can be achieved by interaction with incoherent phonons [7].
The in¬‚uence of interactions on condensate-number ¬‚uctuations in a BEC of microcavity photons was studied in Ref. 8.
The kinetics of photon thermalization and condensation was analyzed in Refs. 9Ђ“11. The kinetics of trapped photon
gas in a microcavity, ¬Ѓlled by a dye solution, was studied, and, a crossover between driven-dissipative system laser
dynamics and a thermalized Bose-Einstein condensation of photons was observed [12].
In previous theoretical studies the equation of motion for a BEC of photons con¬Ѓned by the axially symmetrical
trap in a microcavity was obtained.
It was assumed that the changes of the cavity width are much smaller than
the width of the trap [13]. This assumption results in the coordinate-independent e¬Ђective photon mass mph and
photon-photon coupling parameter g. In this Paper, we study the local super¬‚uid and normal density pro¬Ѓles for
trapped two-dimensional gas of photons with the coordinate-dependent e¬Ђective mass and photon-photon coupling
parameter in a an optical microcavity, ¬Ѓlled by a dye solution. We propose the approach to study the local BEC
and local super¬‚uidity of cavity photon gas in the framework of local density approximation (LDA) in the traps of
larger size without the assumption, that total changes of the cavity width are much smaller than the size of the trap.
In this case, we study the e¬Ђects of coordinate-dependent e¬Ђective mass and photon-photon coupling parameter on
the super¬‚uid and normal density pro¬Ѓles as well as the pro¬Ѓles of the local temperature of the phase transition for
trapped cavity photons. Such approach is useful for the mirrors of smaller radius with the high trapping frequency,
2
which provide BEC and super¬‚uidity for smaller critical number of photons at the same temperature.
The paper is organized in the following way.
In Sec. II, we obtain the condensate density pro¬Ѓle for trapped
microcavity photon BEC with locally variable mass and interactions. The expression for the number of particles in a
condensate is analyzed in Sec. III. In Sec. IV, the dependence of the condensate parameters on the geometry of the
trap is discussed. In Sec. V, we study the collective excitation spectrum and super¬‚uidity of 2D weakly-interacting
Bose gas of cavity photons. The results of our calculations are discussed in Sec. VI. The proposed experiment for
measuring the distribution of the local density of a photon BEC is described in Sec. VII. The conclusions follow in
Sec. VIII.
II. THE CONDENSATE DENSITY PROFILE
While at ¬Ѓnite temperatures there is no true BEC in any in¬Ѓnite untrapped two-dimensional (2D) system, a true
2D BEC quantum phase transition can be obtained in the presence of a con¬Ѓning potential [14, 15]. In an in¬Ѓnite
translationally invariant two-dimensional system, without a trap, super¬‚uidity occurs via a Kosterlitz€'Thouless
super¬‚uid (KTS) phase transition [16]. While KTS phase transition occurs in systems, characterized by thermal
equilibrium, it survives in a dissipative highly nonequilibrium system driven into a steady state [17].
The trap for the cavity photons can be formed by the concave spherical mirrors of the microcavity, that provide
the axial symmetry for a trapped gas of photons. Thus the transverse (along xy plane of the cavity) con¬Ѓnement
of photons can be achieved by using an optical microcavity with a variable width. Let us introduce the frame of
reference, where z€'axis is directed along the axis of cavity mirrors, and (x, y) plane is perpendicular to this axis. The
energy spectrum E(k) for small wave vectors k of photons, con¬Ѓned in z direction in an ideal microcavity with the
coordinate-dependent width L(r), is given by [2]
E(k) =
ЇhЂcњn
...
[23] L. Onsager, ЂњStatistical Hydrodynamics,Ђќ Nuovo Cimento Suppl. 6, 279 (1949).
[24] RP Feynman, ЂњApplication of Quantum Mechanics to Liquid Helium,Ђќ Prog. Low Temp. Phys. 1, 17 (1955).
[25] PC Hohenberg and PC Martin, ЂњMicroscopic Theory of Super¬‚uid Helium,Ђќ Ann. Phys. 34, 291 (1965).
[26] G. Blatter, MY FeigelЂman, YB Geshkenbein, AI Larkin, and VM Vinokur, ЂњVortices in high-temperature super-
conductors,Ђќ Rev. Mod. Phys. 66, 1125 (1994).
[27] NS Voronova and Yu. E. Lozovik, ЂњExcitons in cores of exciton-polariton vortices,Ђќ Phys. Rev. B 86, 195305 (2012);
NS Voronova, AA Elistratov, and Yu. E. Lozovik, ЂњDetuning-Controlled Internal Oscillations in an Exciton-Polariton
Condensate,Ђќ Phys. Rev. Lett. 115, 186402 (2015) .
[28] A. Gri¬ѓn, ЂњConserving and gapless approximations for an inhomogeneous Bose gas at ¬Ѓnite temperatures,Ђќ Phys. Rev. B
53, 9341 (1996).
[29] AA Abrikosov, LP Gorkov and IE Dzyaloshinski, Methods of Quantum Field Theory in Statistical Physics (Prentice-
Hall, Englewood Cli¬Ђs. NJ, 1963).
[30] OL Berman, Yu. E. Lozovik, and DW Snoke, ЂњTheory of Bose-Einstein condensation and super¬‚uidity of two-
dimensional polaritons in an in-plane harmonic potential,Ђќ Phys. Rev. B 77, 155317 (2008).
[31] OL Berman, R. Ya. Kezerashvili, and K. Ziegler, ЂњSuper¬‚uidity and collective properties of excitonic polaritons in gapped
graphene in a microcavityЂќ, Phys. Rev. B 86, 235404 (2012).
[32] A. Amo, J. Lefr`ere, S. Pigeon, C. Adrados, C. Ciuti, I. Carusotto, R. Houdrґe, E. Giacobino, and A. Bramati, ЂњSuper¬‚uidity
of polaritons in semiconductor microcavities,Ђќ Nature Physics 5, 805 (2009).
[33] JP Fernґandez and WJ Mullin, ЂњThe Two-Dimensional Bose€'Einstein Condensate,Ђќ J. Low. Temp. Phys. 128, 233
De las anotaciones necesitamos información
solo sobre autores y subsecciones de artículos.
A continuación, como ejemplo, consideramos una serie de artículos de la sección cond-mat para el año 2017. Todo lo descrito es fácil de reproducir para cualquier otra sección.
La forma más sencilla de comenzar el estudio de textos es hacer una lista de palabras clave de interés para nosotros y calcular la proporción de artículos en los que aparecen.
Permítanos evaluar el cambio en la participación, por ejemplo, en comparación con 2010.
La diferencia en acciones para los años 2017 y 2010. (nota: el Premio Nobel de Física en 2016 fue otorgado por el estudio de las fases topológicas de la materia)Además, según el contenido de los textos, creamos el modelo
word2vec (para la visualización es mejor tener una ventana más amplia, 20 palabras). Para cada clave, tomamos N vecinos más cercanos y con la ayuda de t-SNE calculamos sus coordenadas 2D. Vemos cómo se relacionan las palabras clave entre sí:
Nube de palabras clave y sus satélites, N = 100. Cuanto más lejos esté el enésimo vecino, más se resaltará la palabra. Pares relevantes: Grafeno + Semiconductor, Qubit + Punto, Topológico + Hall, Bose + CondensaciónEn arxiv hay subsecciones para cada sección. Descubrimos en qué subsecciones se encuentran las palabras clave:
Decodificación de los nombres de subdivisiones de cond-matLo anterior describe el trabajo con un conjunto de palabras clave que desea componer manualmente, pero algunos temas pueden haberse omitido. Construyamos un modelo
LDA y veamos los temas:
Para cada tema, obtenemos una lista de artículos correspondientes:
Como sabes, al leer artículos siempre es útil estudiar los enlaces. ¿Podemos recopilar información sobre ellos? Podemos! Miremos la cola del texto.
Cola[23] L. Onsager, ЂњStatistical Hydrodynamics,Ђќ Nuovo Cimento Suppl. 6, 279 (1949).
[24] RP Feynman, ЂњApplication of Quantum Mechanics to Liquid Helium,Ђќ Prog. Low Temp. Phys. 1, 17 (1955).
[25] PC Hohenberg and PC Martin, ЂњMicroscopic Theory of Super¬‚uid Helium,Ђќ Ann. Phys. 34, 291 (1965).
[26] G. Blatter, MY FeigelЂman, YB Geshkenbein, AI Larkin, and VM Vinokur, ЂњVortices in high-temperature super-
conductors,Ђќ Rev. Mod. Phys. 66, 1125 (1994).
[27] NS Voronova and Yu. E. Lozovik, ЂњExcitons in cores of exciton-polariton vortices,Ђќ Phys. Rev. B 86, 195305 (2012);
NS Voronova, AA Elistratov, and Yu. E. Lozovik, ЂњDetuning-Controlled Internal Oscillations in an Exciton-Polariton
Condensate,Ђќ Phys. Rev. Lett. 115, 186402 (2015) .
[28] A. Gri¬ѓn, ЂњConserving and gapless approximations for an inhomogeneous Bose gas at ¬Ѓnite temperatures,Ђќ Phys. Rev. B
53, 9341 (1996).
[29] AA Abrikosov, LP Gorkov and IE Dzyaloshinski, Methods of Quantum Field Theory in Statistical Physics (Prentice-
Hall, Englewood Cli¬Ђs. NJ, 1963).
[30] OL Berman, Yu. E. Lozovik, and DW Snoke, ЂњTheory of Bose-Einstein condensation and super¬‚uidity of two-
dimensional polaritons in an in-plane harmonic potential,Ђќ Phys. Rev. B 77, 155317 (2008).
[31] OL Berman, R. Ya. Kezerashvili, and K. Ziegler, ЂњSuper¬‚uidity and collective properties of excitonic polaritons in gapped
graphene in a microcavityЂќ, Phys. Rev. B 86, 235404 (2012).
[32] A. Amo, J. Lefr`ere, S. Pigeon, C. Adrados, C. Ciuti, I. Carusotto, R. Houdrґe, E. Giacobino, and A. Bramati, ЂњSuper¬‚uidity
of polaritons in semiconductor microcavities,Ђќ Nature Physics 5, 805 (2009).
[33] JP Fernґandez and WJ Mullin, ЂњThe Two-Dimensional Bose€'Einstein Condensate,Ђќ J. Low. Temp. Phys. 128, 233
PDF Converter maneja bien una sección con una bibliografía. Esto significa que los enlaces se pueden recuperar con algunas expresiones regulares. Como resultado, obtenemos una lista de artículos que se deben leer con frecuencia.
Consulte estos enlaces en
Google Scholar :
Haga una lista de los autores más activos para cada subsección: extraemos y calculamos el contenido de la etiqueta de autor a partir de las anotaciones. El número de artículos publicados por un autor en particular es una característica comprensible, pero poco confiable, puede complementarse con la mediana del número de coautores (ver cuadernos).
Los autores de mes-hall no tienen rival: su ritmo de trabajo promedio es de más de un artículo por semana ...Finalmente, estimamos la proporción de subsecciones:
Portátiles de demostración:
cond-mat.17 ,
astro-ph.17 ,
cs.17 ,
math.17Github :
ilovescience